1. A simply-supported steel beam has a diameter of 1.25 in, and design requirements demand that the deflection in any direction at x = 10 in should be less than δ = 0.00375 in. Find the probability of failure given that P_z ~ N(100,8²) lb and P_y ~ N(200,10²) lb using Monte Carlo Simulation. Note that P_z and P_y are independent.

Solution

Step 1: Define the limit-state function.

              Limit-state function is then given by

, where .

              Therefore,

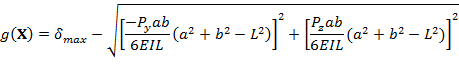
where  is the distance to forces from origin,   is the length of the beam, ,  is the Young's modulus of steel, and  is the moment of inertia about y or z axis.

Step 2: Sample the random variables.

Step 3: Evaluate the limit-state function.

Step 4: Analyze statistically.

Failure occurs when .

Define an indicator function

Sum the number of failures

Probability of failure

Number of Failures:      248092

Number of simulations:  1.000e+07

Probability of failure: 0.0248092 Ans.