58. A torsion bar OA is fixed at O and is simply supported at A. It is connected to a cantilever AB, and a force  is applied at B. Bar OA has a spring rate of  and a length of . Cantilever AB has a spring rate of  and a length of . If the allowable deflection at B is , determine the probability of failure using First Order Second Moment Method. Assume that ,  and  are independent.

Solution

The torque of torsion bar OA is

Thus the angle of twisting is

Then the deflection  at B resulted from the twisting of torsion bar OA is

For the cantilever BC, the deflection  at B resulted from force P is

Then the overall deflecting at B is

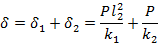
The limit-state function is the actual deflection subtracted from the allowable deflection. Failure occurs when.

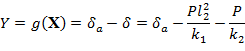
where .

Using FOSM, we have

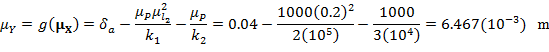
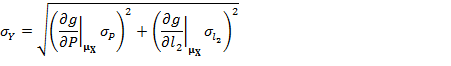
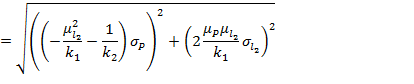
The probability of failure is then given by

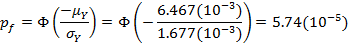
Ans.