Example 1

The position of a slider-crank mechanism is required to be  mm when . If the actual position  is outside the tolerance range  mm, it is considered that a failure occurs. The design variables are independent and follow normal distributions of , , and . Use the First Order Second Moment (FOSM) method to calculate the probability of failure.

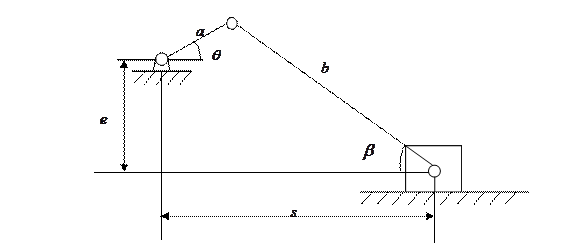
Solution:

The vector of random variables is . The actual position of the slider is given by

Since the tolerance range is , failure occurs when  or . Let , and . Then, the probability of failure of the mechanism is given by

Since the design variables are independent and follow normal distributions,  and  also follow normal distributions with  and , respectively.

According to the FOSM method, the mean and standard deviation of  are calculated by

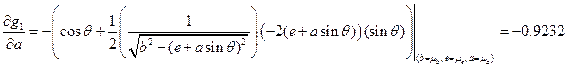
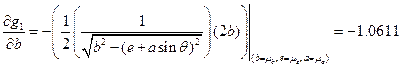
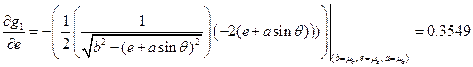
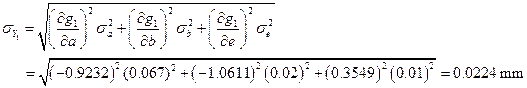
Thus, we have

Similarly, the mean and standard deviation of  are calculated by

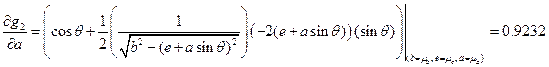
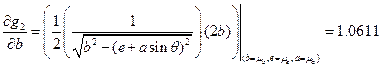
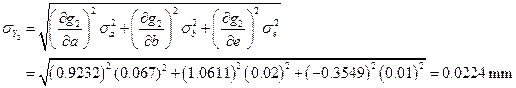
We have

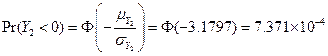
The probability of failure of this mechanism is then given by

Ans.